Free Energy and Equilibrium
A negative value of DG implies that a reaction is
product-favored, i.e., after the reaction is completed there are more products
than reactants.
Conversely,
a positive value of DG implies that a reaction is
reactant-favored, i.e., after the reaction is completed there are more reactants
than products.
In the Equilibrium Tutorial, the equilibrium constant, K, was discussed, which related the amounts of products and reactants of a reaction at equilibrium. A large value of K implies larger amounts of products than reactants, while a small value of K implies larger amounts of reactants than products.
Since both DG and K predict the relative amounts of reactants and products after a reaction is over, you may be wondering if DG and K are somehow related to each other. This is indeed the case:
DGº = -RTlnKth (2)
where R is the gas constant (8.314 J/mol•K), T is the temperature in Kelvins, and Kth is the thermodynamics equilibrium constant (equal to Kc and related to Kp by an equation described in the Equilibrium Tutorial). Thus, if Kth > 1, lnKth > 0 and the minus sign means that DGº < 0, implying a product-favored reaction. If DGº > 0, lnKth < 0 and Kth < 1, implying a reactant-favored reaction, as discussed above.
In practice, equation (2) is used if either DGº or Kth are difficult to measure directly. For example, the reaction of hydrogen and oxygen gases to form water vapor:
2H2(g) + O2(g) 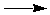 2H2O( 2H2O(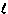 ) )
is so product-favored that it is impossible to measure the concentrations of hydrogen and oxygen gases to calculate Kth directly. Instead, DGº can be calculated from DGfº values and the value of Kth can be calculated from equation (2).

 Calculate the value of Kth for the reaction of hydrogen and oxygen.
Calculate the value of Kth for the reaction of hydrogen and oxygen.
|